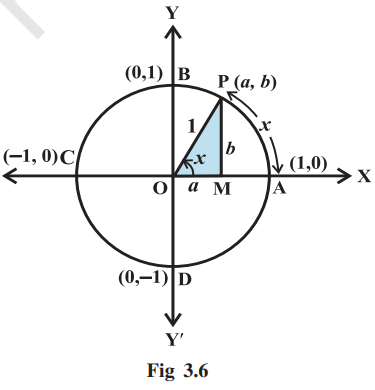
Consider a unit circle with centre at origin of the coordinate axes. Let P (a, b) be any point on the circle with angle AOP = x radian, i.e., length of arc AP = x
Trigonometric Functions
We define cos x = a and sin x = b Since ∆OMP is a right triangle, we have OM2 + MP2 = OP2 or a2 + b2 = 1 Thus, for every point on the unit circle, we have a2 + b2 = 1 or cos2 x + sin2 x = 1 Since one complete revolution subtends an angle of 2π radian at the centre of the circle, ∠AOB = π / 2
∠AOC = π and ∠AOD = 3π 2 . All angles which are integral multiples of π / 2 are called quadrantal angles. The coordinates of the points A, B, C and D are, respectively, (1, 0), (0, 1), (–1, 0) and (0, –1). Therefore, for quadrantal angles, we have
cos 0° = 1 sin 0° = 0,
cosπ / 2 = 0 sinπ / 2 = 1
cosπ = − 1 sinπ = 0
cos3π/ 2 = 0 sin3π / 2 = –1
cos 2π = 1 sin 2π = 0
Now, if we take one complete revolution from the point P, we again come back to same point P. Thus, we also observe that if x increases (or decreases) by any integral multiple of 2π, the values of sine and cosine functions do not change. Thus
sin (2nπ + x) = sin x, n ∈ Z , cos (2nπ + x) = cos x , n ∈ Z
Further, sin x = 0, if x = 0, ± π, ± 2π , ± 3π, …, i.e., when x is an integral multiple of π and cos x = 0, if x = ± π / 2 , ± 3π/ 2 , ± 5π /2 , … i.e., cos x vanishes when x is an odd multiple of π/ 2 . Thus
sin x = 0 implies x = nπ, where n is any integer
cos x = 0 implies x = (2n + 1) π / 2 , where n is any integer.
other trigonometric functions
cosec x = 1/ sin x , x ≠ nπ, where n is any integer
sec x = 1 /cos x , x ≠ (2n + 1) π /2 , where n is any integer
tan x = sinx /cos x , x ≠ (2n +1) π /2 , where n is any integer.
cot x = cosx / sinx , x ≠ n π, where n is any integer
We have shown that for all real x, sin2 x + cos2 x = 1
1 + tan2 x = sec2 x
1 + cot2 x = cosec2 x
Table of values of trigonometric ratios for 0°, 30°, 45°, 60° and 90°
| 0° | π/6 | π/4 | π/3 | π/2 | π | 3π/2 | 2π | |
|---|---|---|---|---|---|---|---|---|
| sin | 0 | 1/2 | 1/√2 | √3/2 | 1 | 0 | -1 | 0 |
| cos | 1 | √3/2 | 1/√2 | 1/2 | 0 | -1 | 0 | 1 |
| tan | 0 | 1/√3 | 1 | √3 | undefined | 0 | undefined | 0 |
